Ch. 9 final review. Go time.

Hello.
First off, some background music. (Press control N, then click on it to play it in the background)

Alright, chapter 9 was all about triangles with one exception:
Brahmagupta's formula.
This states that any cyclic quadrilateral (and quadrilateral that can be inscribed in a circle) can have it's area calculated by taking half the perimeter (s) and finding the value of the radicand (s)(s-a)(s-b(s-c)(s-d).

This is a play off of Heron's formula, in which the area of ANY triangle can be found by taking the value of
these theorems require you to know all of the side lengths. (SSS)


 Then we had that wonderful little add on of the law of sines and cosines.

Law of sines:       a       +       b      +        c       = Area
                       sinA           sinB             sinC
This requires knowing a side and it's optional angle. (SSA, AAS)

Law of cosines: this took a really long time to derive in class I remember.

­a­²₌ b²+c²-2bcCosA

Law of cosines works with SAS.

Okay, now for the easy part.

angle bisector theorem:

A picture is worth a thousand words, is it not?

With any right triangle, you can drop an altitude that goes through a vertex and all the triangles now formed are similar. You can then use geometric means to figure out sides.

Por ejemplo, if AB=6, and AD=3, we can set up a proportion using AB as the geometric mean.

AB= x 

AD  AB

So we have 3x=36

x=12.

The pictures make it easier to read.

Side splitter. Here we go.

Just proportions. The triangle formed by the sidesplitter (sounds like a sick wrestling move, doesn't it?) is similar to the original. The ratio of areas of these triangles is 1:9. So, VX=8.
 Also, these triangles are similar because they have the mutual angle and the parallel lines do the rest.

I think it safe to assume you all know about the basics of circles, the pythagorean theorem, about the multiplication and addition of radicals, and distance formula, but here you go.

Oh wait! 30-60-90 right triangles always have side ratios that a=s b=s(root)3 and c=2s.

45-45-90 right triangles have congruent a and b=s, and c=s(root)2.

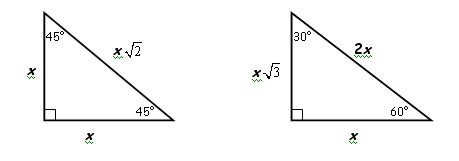
Oh, wait! Trigonometry!

so, by taking the tangent, ground distance, and angle of elevation, you can find how far away the lighthouse is from this man's head! Important to all you light house philosophers.

This also works for the angle of depression, the only difference is you use the angle of depression instead of the angle of elevation.


This blog post is quite the keyboard full.



 that's a lot said with a little.


Sweet Caroline, I think that's everything.

slant height, a'ight?

Wow. This is only a small fraction of what we've learned with our beloved teacher Mr. Wilhelm educating us and challenging us to prepare us for the future. Glad to be out of my two hardest classes, but I'm going to miss Mr. Wilhelm. He has been a great inspiration to us all.











We love you Mr. Wilhelm.

I'm off.
-Shane
P.S. Don't let anyone but yourself decide who you will become.